
Substitute this into the integral, we have Let us see an example and solve an integral using this antiderivative rule. The antiderivative chain rule is used if the integral is of the form ∫u'(x) f(u(x)) dx. The chain rule of derivatives gives us the antiderivative chain rule which is also known as the u-substitution method of antidifferentiation. We know that antidifferentiation is the reverse process of differentiation, therefore the rules of derivatives lead to some antiderivative rules. Please do not confuse this power antiderivative rule ∫x n dx = x n+1/(n + 1) + C, where n ≠ -1 with the power rule of derivatives which is d(x n)/dx = nx n-1. Using the antiderivative power rule, we can conclude that for n = 0, we have ∫x 0 dx = ∫1 dx = ∫dx = x 0+1/(0+1) + C = x + C.

Let us consider some of the examples of this antiderivative rule to understand this rule better. This rule is commonly known as the antiderivative power rule. Now, the antiderivative rule of power of x is given by ∫x n dx = x n+1/(n + 1) + C, where n ≠ -1.

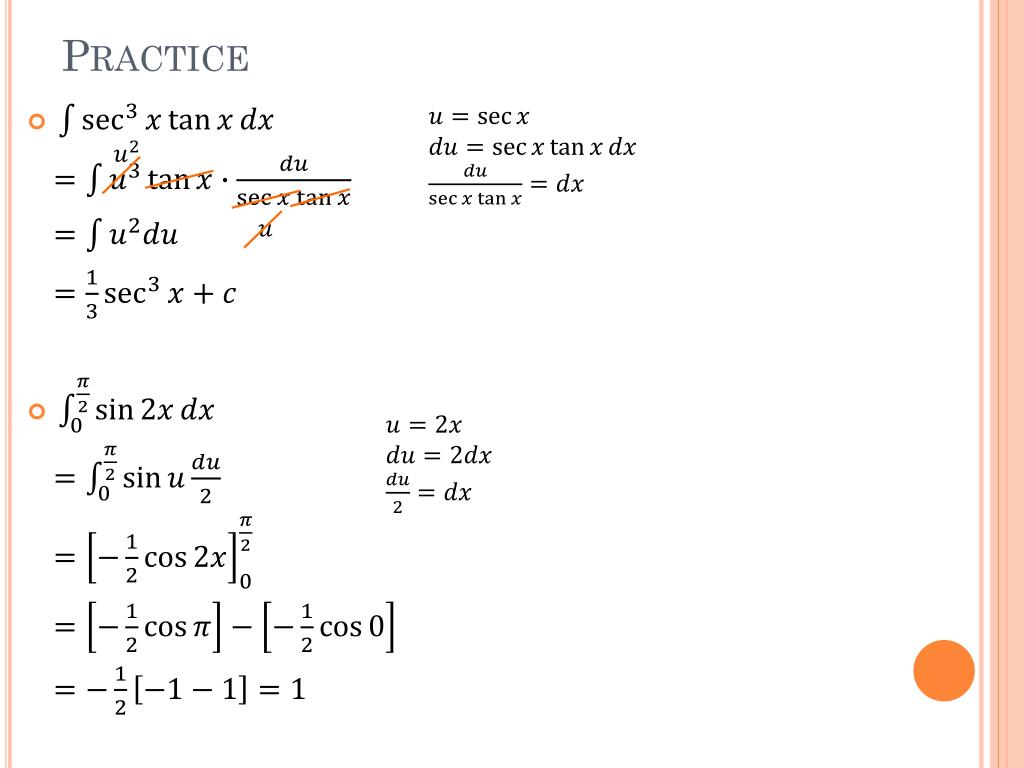
The antiderivative rules are common for types of functions such as trigonometric, exponential, logarithmic, and algebraic functions. We will discuss the rules for the antidifferentiation of algebraic functions with power, and various combinations of functions. It helps to find integration of a complex function with a direct and easy method.In this section, we will explore the formulas for the different antiderivative rules discussed above in detail. It is known as reverse chain rule or u-substitution or substitution rule. Yes, there is a technique of finding integration by using chain rule in integration.
b∫ a f(t) dt = F(b) - F(a) (integration of definite integrals). d / dx x∫ a f(t)dt = f(x) (derivative of indefinite integrals). One rule is to find the derivative of indefinite integrals and the second is to solve definite integrals. The fundamental theorem of calculus defines two rules to solve integration. This rule is also known as the substitution method. It is used to solve those integrals in which the function appears with its derivative. There is a chain rule in integration also that is the inverse of chain rule in derivatives. Related: Is Chain Rule same as Product Rule? - Difference & Comparison FAQ’s Does Chain Rule exist in Integration? It calculates the antiderivative of a function by using its derivative. It calculates the rate of change of a function with respect to another function. The reverse chain rule formula for a function Suppose for a function y = fgx the chain rule formula can be written as, This rule can also be called the “substitution Rule", or the “U-Substitution Rule". The reverse chain rule combines these two parts of the function and integrates it directly. We can do the reverse of chain rule to integrate complicated functions where the function and its derivative appear in a combined form. It is a technique that allows us to find derivatives. Since the chain rule is used for derivatives to calculate derivative of complex functions or the function in combination form. The reverse chain rule is a technique of finding integration of a function whose derivative is multiplied with it. This article will help you to understand the reverse chain rule and the difference between the chain rule in derivative and integration. Same as derivatives, there is a chain rule in integration known as the reverse chain rule. In derivatives, we have a chain rule to evaluate derivatives of complex functions. Is there a chain rule for integration? Introduction







 0 kommentar(er)
0 kommentar(er)
